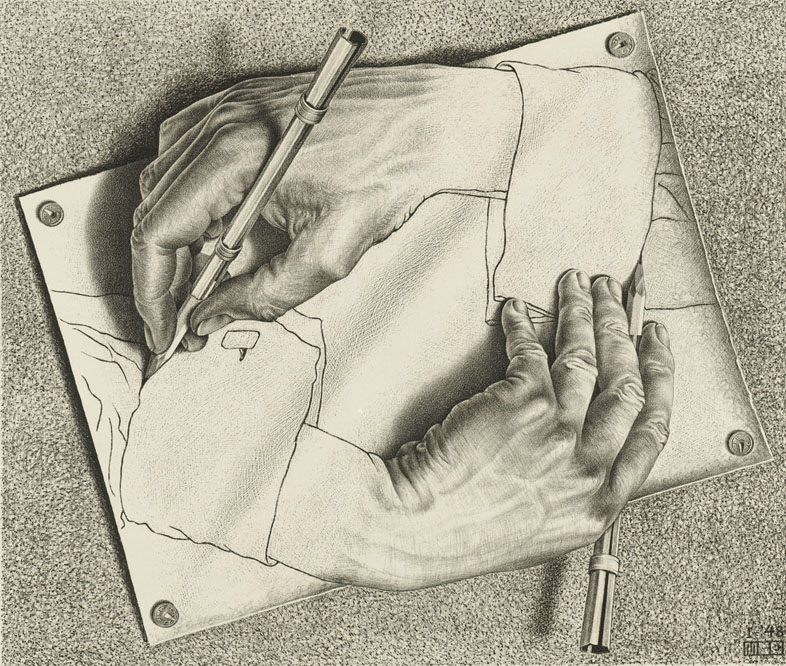
Like Gustav Klimt or Claude Monet, the unwilling darlings of dorm room posters everywhere, Maurits Cornelis Escher belongs to the class of meritorious artists whose cult followings do them no reputational favors. Escher’s meticulous constructions sit at the intersection of popular accessibility and aesthetic merit. To the extent that he was courted by elite academic circles, he was acclaimed by mathematicians and scientists, not critics or aesthetes: Escher work beguiled scholars at the 1954 International Congress of Mathematicians, whose organizers arranged an exhibition at the nearby Stedelijk Museum, and, six years later, he presented to resounding applause at the Fifth International Congress of Crystallography at Cambridge.
Academic popularity notwithstanding, the unassuming Dutch artist has remained something of a stranger to more conventional artistic venues. Only one of his works is on display in a public collection in Britain (purchased by the geography department at Glasgow University and later re-hung in the campus art museum), and his first major retrospective occurred when he was seventy years old – by which time he had already sold more than six-hundred prints of a single woodcut and declined Stanley Kubrick’s invitation to design sets for 2001: A Space Odyssey. (Four years later, he would issue a similar rebuff to Mick Jagger, who had the gall to address him as “Maurits” in a letter requesting permission to use his work as an album cover. Escher replied via Jagger’s assistant, “Please tell Mr Jagger I am not Maurits to him.”)
Too stiff to embrace the psychedelic culture clambering to adopt him, Escher was never fully at home in any of the milieus that claimed him. His aesthetic orientation was anathema to the narrowly functional atmosphere that prevailed at the School of Architecture and Decorative Arts in Haarlem, where he studied graphic design from 1919-22, but his work proved excessively technical for connoisseurs of the finer arts. He never gained more than partial membership to any of the traditions with which he flirted over the course of his career.
It is not surprising, then, that The Amazing World of M.C. Escher, the first major show of Escher’s work in the United Kingdom, aspired to re-canonization. “We hope that this exhibition will go some way towards reassessing his work,” the curators declared in their forward to the catalogue. The exhibition, displayed in Dulwich Picture Gallery until early 2016, attempted to situate Escher more comfortably in the critically sanctioned pantheon. But, to its credit, the show refrained from smoothing over the tensions that characterize Escher’s work along with his institutional position, producing a series of frictions that might have pleased an artist with a lifelong fixation on paradox.
Escher’s favorite perplexity was representation, something he considered to be intrinsically concerned with radical differences and reconciliations. He understood that artists deal in transmutations, including that of two-dimensional materials into the illusion of three-dimensional spaces, lines into objects, colors into textures. Escher dabbles in this representational alchemy with an eye to exposing its workings. In Metamorphosis II (1940), perhaps the most recognizable of the Metamorphosis woodcuts, a series of regular patterns gradually morph from shapes into animals and back again. A grid becomes a mass of lizards, which devolves into a honeycomb that in turn gives way to bees. The boundary between abstract form and meaningful content proves slippery, as fish become birds and birds become cubes stripped of any figural significance. Representations, Escher suggests, are not accidents but deliberate transfigurations: imagistic rudiments must be artificially molded into legible signs. Metamorphosis II undermines the usual order of artistic construction, yielding representations that threaten at each moment to revert to their primitive origins.
Even in Escher’s earlier and less obviously conceptual drawings the artist adamantly insists on artificiality, imposing a jarringly deliberate order onto the natural world. In the 1921 woodcut Paradise, Eden is a domain of absolute symmetry. Adam and Eve stand stiffly in the center of the work, and the mountains that loom behind them arch into a series of sharp, perfectly formed triangles. Eden figures in our cultural mythology as an idyll of natural flourishing, but here the legendary garden succumbs wholesale to Escher’s rigid organizational schema. The vista depicted in another early work, Bonifacio, Corsica (1928), boasts a similarly geometric geography: austerely rectangular buildings cluster atop a cliff whose sheer face is regularly patterned, as if it had been constructed. This is an architect’s landscape, built rather than chronicled.
Much later, in his so-called “impossible object” drawings, Escher would reverse the paradigm he advanced in these early efforts: when he tired of representing the real world unrealistically, he crafted realistic depictions of unrealistic spaces. In the aptly titled lithograph Up and Down (1947), a staircase seems to spiral in both directions simultaneously, allowing a morose schoolboy seated on the steps to gaze both up and down at a chastising maternal figure on the balcony. Although this scene is more realistically rendered than Paradise or Escher’s sketches of Corsica, it too establishes palpable friction between the natural and the artistic: its form, which is believable, and its content, which is inconceivable, co-exist in subtle disagreement.
The stair theme, a favorite of Escher’s, recurs in House of Stairs (1951), Relativity (1953), and Convex and Concave (1955). These drawings, which invariably return would-be voyagers to their points of origin, are depressingly cyclical: in House of Stairs, larvae-like creatures scuttle up a flight of stairs that winds back around, only to deposit them anew at the foot of their original ascent. In Relativity, we regard a large manor from several angles at once. Faceless humanoid figures ascend stairs that seem to lead every way but out. Their trajectories run parallel to another, locking the work’s inhabitants into a series of dead ends from which they are unable to effect contact or intersection.
Though Escher’s tessellations (patterns featuring recurring figures) represent forays into the same sorts of infinite, inescapable regressions, their tenor often proves more optimistic. Like the stair drawings, Encounter (1944) rejects the idea of linear progress – but unlike Relativity, it insists on the inevitability of interaction, even violent collision. Another cyclical study, the work depicts a ring of upright dancers who gradually transform into stooped demons, then metamorphose back into their forebears. At the center of the lithograph, the circle comes to a head when a demon and a dancer clasp hands in acknowledgement of their bond. Encounter unites two extreme ends of the same spectrum: extreme abstraction (Escher treats bodies as shapes, fitting them into each other) and extreme familiarity (his configurations make use of animals and humans, not impersonal geometric figures).
Throughout Escher’s canon, tessellations underscore the curious relationship of specificity to generality, of finite shapes to infinite spaces. “In certain systems, a plane can be filled or divided into infinity, with various and increasingly complex geometric figures that nevertheless conform to the following rule: the figures are congruent and fill the space through rhythmic repetition, leaving no empty spaces. When one seeks to fit such figures together in such a manner that they arouse within the viewer associations with something familiar, such as an object or a living creature, then a fascinating game emerges, because it affords the artist infinite possibilities yet confines him to the limitations imposed by the rules of play,” he wrote in 1947. Escher’s infinities are comfortably tempered by repetition: the patterns he selects can continue on forever in the same way, but they negate the enormity of infinity by rendering it consistent and predicable.
Disorder, taken to its logical extreme, results in a kind of order. But by the same token, Escher’s precise organizations devolve into the madness of his impossible object scenes, which use mathematical terminations to reach dreamlike, fantastical ends. Escher’s works do and do not reconcile the series of contradictions they evoke. Instead, they show that a bird can easily become a fish, that one appearance can easily coincide with another. The results frustrate the cherished but curmudgeonly conviction that playfulness prohibits rigor.